What is a Tolerance Level?
Tolerance Intervals vs. Confidence Intervals
One way to understand the tolerance level statistic is to compare tolerance intervals to confidence intervals. Let’s use the example of height of people as the parameter of interest and the people living in Beaverton as the population of interest (sampled population).A confidence interval calculated from a sample of people living in Beaverton would give an average height of people in Beaverton, plus or minus a certain value for height to reflect the variability in expected future samples (e.g. 5.8 ft + 0.6 ft). For example, a 90% confidence interval with an alpha of 0.05, would be interpreted as follows: If we repeatedly sample the heights of people in Beaverton, we could be 95% certain that 90% of the average heights from the samples would fall within the interval from the original sample (5.2 to 6.4 ft). We can predict this based on the mean and variability of the sample.
A tolerance interval is similar to a confidence interval but with a key difference: tolerance intervals are estimates of the percent of all individuals in the population that are within some specified range of values. Based on the mean and variability of the sample, we can be 95% certain that 90% of the individual heights in the population (not sample means as above) would fall within the interval if the whole population were sampled. In our example above, the tolerance interval indicates that if the height of every individual in Beaverton were measured, we could be 95% certain that 90% of all the individuals in Beaverton would have a height within the interval of 5.2 to 6.4 ft.
Tolerance Levels in the DecAID Advisor
In the DecAID Advisor tolerance levels are used. Levels are one-sided intervals with the lower limit of the interval being zero. Thus, an 80% tolerance level indicates 80% of the individuals in the population have a value for the parameter of interest between 0 and the value for the 80% tolerance level. Or conversely, 20% of the individuals in the population have a value for the parameter of interest greater than the 80% level. An alpha level of 0.10 was used when calculating the tolerance levels. For example, an 80% tolerance level of wildlife use of snag diameter (dbh) means that 80% of all individuals observed of some species (combined across one or more wildlife studies) uses snags less than or equal to some specifiec dbh, and 20% use snags greater than that dbh.Wildlife Data
We calculated three tolerance levels (30%, 50% and 80%) for dbh of snags and diameter of down wood used by wildlife species, and density of snags and percent cover of down wood in areas used by wildlife species. With normally distributed data the 50% tolerance level is simply the mean. See the Statistical Basis for DecAID document for details of the actual calculations used.Here is an example. Using the parameter of nest snag dbh for pileated woodpeckers from the Eastside Mixed Conifer Forest, Larger trees (EMC_L) wildlife habitat type, we can say (with 90% certainty) that in the EMC_L vegetation condition:
-
· 30% tolerance
level = 64.5 cm (25.1 in), thus, 30% of nest snags used by pileated woodpeckers
are <
64.5 cm dbh and 70% of nest snags used by pileated woodpeckers are > 64.5
cm dbh
· 50% tolerance level = 74.8 cm (29.2 in), thus, 50% of nest snags used by pileated woodpeckers are < 74.8 cm dbh and 50% of nest snags used by pileated woodpeckers are > 74.8 cm dbh
· 80% tolerance level = 90.6 cm (35.3 in), thus, 80% of nest snags used by pileated woodpeckers are < 90.6 cm dbh and 20% of nest snags used by pileated woodpeckers are > 90.6 cm dbh
Inventory Data
The inventory tolerance levels are calculated also at 30%, 50%, and 80% using the same certainty level of 90% applied to the wildlife data. This makes the inventory data directly comparable to the wildlife data. However, the inventory tolerance levels are calculated differently, using a non-parametric method (based on the binomial distribution and sample size) that doesn’t require assuming the dead wood data are normally distributed. See the Statistical Basis for DecAID document for details.With the inventory data, the sample is the set of inventory plots and the population is the total landscape represented by the sample of plots. The inventory tolerance levels describe sizes and amounts of dead wood at the population level, not just for the inventory plot sample. In other words, the tolerance levels describe dead wood conditions across the total area within the vegetation condition.
For the inventory plot data, the tolerance levels describe proportions of the area within a given vegetation condition that have specific sizes or amounts of dead wood. The 50% tolerance level more closely represents the median value than the mean, but the median describes the sample whereas the 50% tolerance level describes the entire population. This is easiest to understand using an example. From the following box-and-whisker diagram for snags >25.4 cm on n=241 unharvested plots in Westside Lowland Conifer-Hardwood Forest, Oregon Cascades, Larger trees (WLCH_OCA_L) vegetation condition.
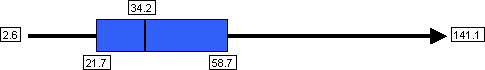
For the WLCH_OCA_L vegetation condition, we can say (with 90% certainty) that:
-
· 30% of the
area in this vegetation condition has <21.7
snags/ha; 70% has > 21.7 snags/ha
· 50% of the area in this vegetation condition has < 34.2 snags/ha; 50% has > 34.2 snags/ha
· 80% of the area in this vegetation condition has < 58.7 snags/ha; 20% has > 58.7 snags/ha
· Snag density ranges from 2.6 to 141.1 snags/ha across this vegetation condition